Texas Hold'em Math 03

Calculating Percentages

All right, now you're ready to learn the percentages.

Using the number of outs you have in a situation, you can quickly calculate your PERCENTAGE OF WINNING the hand.

Below is the chart that I use to calculate all the odds at the poker table.

At first, this chart looks pretty intimidating. But once you learn to use it you'll find it to be quick, easy, and efficient. We'll go through each segment of the chart… but for now, I just want you to pay attention to the left side, under the title "Probability".

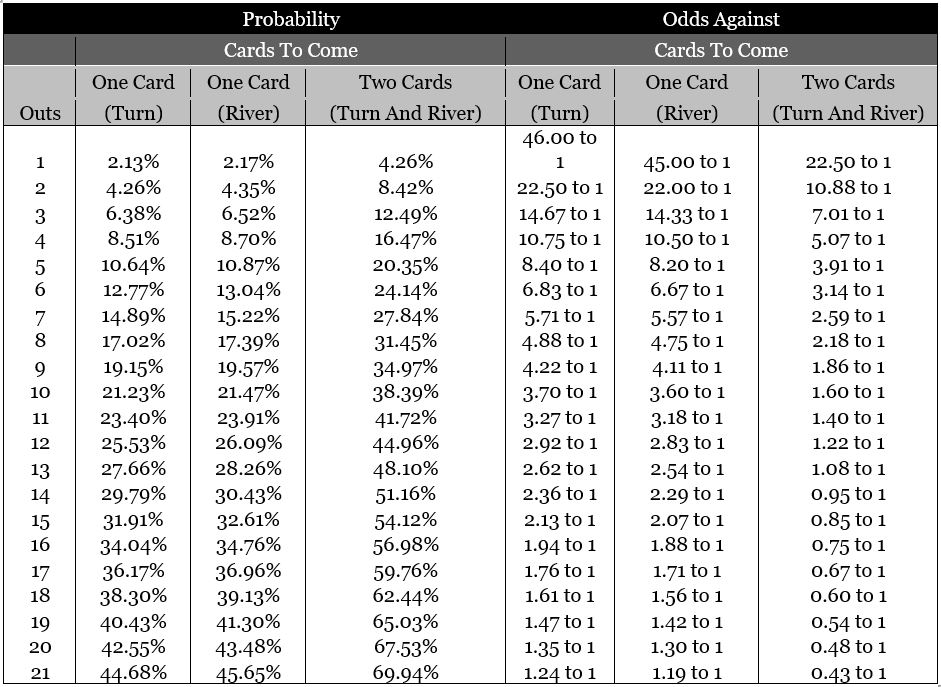
The percentage numbers are the probability that you will catch one of your OUTS.

For instance, let's say you're on a club flush draw after the flop. One more club will give you the flush. The number of OUTS you have is nine (since there are thirteen clubs in the deck and you're already using four of them).

To figure your percentage, just take a look at the chart and find the corresponding row and column. The ROW would be the one that says nine outs. The COLUMN would be "One Card (Turn)"… since you know the flop and have the turn card to come.

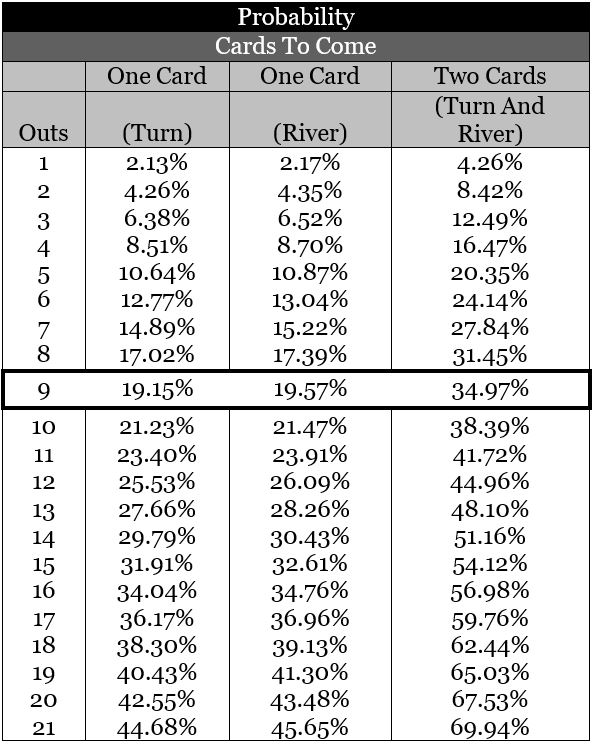
So your percentage chance of getting your flush on the TURN CARD is 19.15%. For the river, it would be 19.57%. This is found by following the same row, but using the next column called "One Card (River)".

The final number in this row is 34.97%. This is the percentage chance you have of making your flush on EITHER the turn or river. As we'll learn, this number is NOT as important as you'd think. It's not used to calculate pot odds.

You might be wondering why the odds for the turn aren't the same for the river. The reason is because the percentage is the number of outs divided by the number of "unknown" cards. After the turn card comes out, there's one less "unknown" card, which means the percentage on the river is slightly higher.

You also might be wondering why the turn card column and river card column don't add up to equal the turn and river card column. For instance, 19.15% plus 19.57% doesn't equal 34.97%. Why is that?

The answer is related to some complicated math. But for the curious, here's a quick example that makes it easy to remember why...

Pretend you have a coin. You're going to flip it twice, and want to know the odds of making "heads". For the first flip, your odds are 50%. For the second flip, your odds are 50%. But what are the odds you'll make it EITHER the first or second time?

If you add 50% plus 50% you'd get 100%, but obviously that's wrong... since there's always the chance of flipping tails twice in a row.

The REAL answer is 75%. This is figured by taking the odds AGAINST making heads for the first flip (1/2) multiplied by the odds AGAINST making odds on the second flip (1/2). That number (1/4) is then subtracted from one to give you ¾, or 75%.

Why is it figured that way?

I don't know and I don't want to know. Who cares? It has nothing to do with poker, so let's get back to the percentage charts so that you can win some more pots.

Let's do another scenario for calculating outs and percentages.

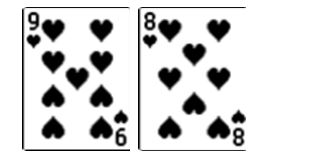
You get dealt suited connectors:

The flop comes out:

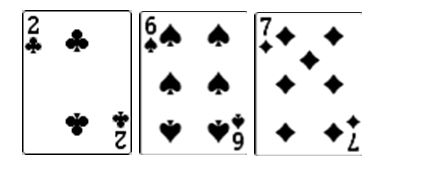
This gives you two over cards and an open-ended straight draw. You don't put anyone on a pocket pair or two pair yet… so what are your odds of having the "winning" hand on the turn? In other words, what's the percentage you'll make one of your "outs" on the turn?

See if you can figure it out on your own right now.

OK, now for the answer. Step one is to calculate the outs. These are the cards that can help you:

4 fives + 4 tens + 3 nines + 3 eights = 14 outs

The eights and nines are over cards, which means getting one of them will give you top pair. Top pair isn't necessarily a winner, but we'll treat it like a winner for our purposes here.

Now it's time to use the probability charts.

With thirteen outs, you've got a 29.79% chance of making your hand on the turn, a 30.43% for the river, and a total chance of 51.16%.

If you only consider the open-ended straight draw for your outs (and not top pair), you'd have eight outs. That means you'd have a 17.02% chance on the turn, 17.39% chance on the river, and a 31.45% chance for both the turn and river taken together.

Get it?

OK, let's do one more example. Except this time let's calculate the odds that you'll LOSE a hand… based on your "read" of the opponents at the table.

Let's say you're dealt pocket Queens:

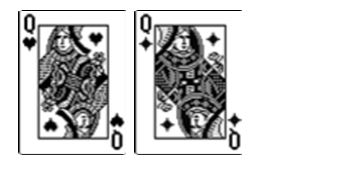
The flop comes out:

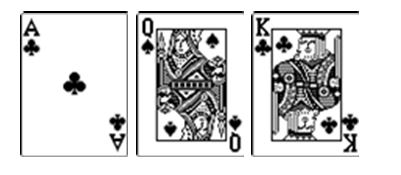
Let's say you pick up a read on your two remaining opponents in the hand. You think one of them has an Ace. You think the other is on a club flush draw.

Using that information, what are the odds your opponents will MAKE their hands (catch their outs) and beat you?

Well, the flush draw has eight outs (the nine remaining clubs minus the Queen of clubs in your hand). Using the percentage charts you'll see that there's a 31.45% chance that opponent will make his hand (on either the turn or river). The other opponent needs a runner-runner situation to stay alive. If another Ace comes out he'll have trips, but that will give you a winning full house.

We'll ignore the runner-runner calculation for now, since it's relatively insignificant. I'll give you the steps to calculating it later.

Ok, so ROUGHLY speaking, you've got a 68.55% chance of winning so far. The turn card comes:

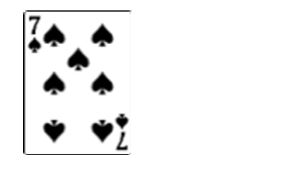
This doesn't help your opponents, but it actually helps YOU. The reason is because now the seven of clubs (which gives your opponent the flush) would pair the board and give you the full house. Since full house beats a flush, your opponent on the club flush draw has now lost an out. He's down to seven outs.

Using the charts you'll see that means your opponent has a 15.22% chance of winning on the river. That gives you a 84.78% chance of winning.

The river comes out:

Your opponent has the flush so he goes all-in. You call with your full house, Queens full of sevens, and win a monster pot.

OK, so that's how you use the odds percentage charts. These charts are very useful for learning more about probability in poker, and can be used any time you play online poker.

For "offline" poker, these charts aren't quite as useful, since you can't carry them around. At the end of this report, after you learn how to calculate betting odds and make pot odds comparisons, I'll show you the SHORTCUTS for figuring out percentages WITHOUT these charts.

There's a simple, easy shortcut you can use to INSTANTLY know the percentages in your head based on a given number of outs. You'll love it.

We'll also talk more about why the column for the turn plus river percentage is rarely used in calculating pot odds.

And of course, we'll tie everything together by giving you PRACTICAL applications of all this knowledge.

But for now, let's keep working on the "foundation" and go over how to calculate runner-runner odds…